Medical Physics: Light

1.

Light is a radiation. It starts at a source (the sun or a lamp) and radiates at enormous speed (about 300,000 km/sec), from that source, in a straight line.

2.

Light consists of photons; these are very small packets of energy (smaller than electrons).

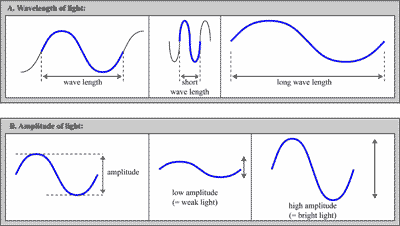
3.

The photons travel as WAVES or ‘wiggles’. These waves can be very fast (short wavelength) or very long (long wavelength).

4.

It is important to note that these photons travel though vacuum; they don’t need a medium for their transportation (unlike sound propagation).

5.

The wavelengths can be very very short such as X-rays and gamma rays (1 nanometer (=nm) meter or less (nm = nanometer = 10-9 m =  0.000000000001).

6.

The wavelength can also be quite long, such as radio waves; typically several meters long.

7.

Our eyes are only sensitive to a small range (or spectrum) of light; from 400 to 700 nm. We see the short wavelength (at about 400 nm) as violet/dark blue, while the longer wavelength (approximately 700 nm) is seen as red. All colours are determined by their wavelengths within the visible range.

8.

The amplitude of the light wave determines the brightness of light. A high amplitude is a very bright light. A low amplitude makes the light dimmer.

9.

Note that the color of the light is determined by the wavelength, not by its amplitude. A red light can be very bright (= high amplitude) or very soft (= low amplitude).

1.

Interesting and useful things happen when light rays crashes into an object, often called a medium.

2.

If the medium is very thin, like gas or air, which has a low density, nothing much will happen to the light rays.

3.

If, on the other hand, light crashes into a high-density medium, like a wall or a table, then some of the light is absorbed (as heat) and some is reflected.

4.

If the wavelength of the reflected light falls within our visible spectrum, then we see a color. So, if a table is red, this is because it absorbs all kinds of light rays and reflects the red light rays.

5.

If all light rays are absorbed, then the object looks black.

6.

If all light rays are reflected, the object looks white.

1.

It becomes more interesting when light is bounced of a mirror. With a good quality mirror, all light rays are bounced back (=reflection).

2.

As seen in the diagram, this bouncing back is determined by the angle between the incoming light rays and the surface of the mirror; this is called the angle of incidence.

3.

The angle of bouncing back (= the angle of reflection) is the same (equal) to the angle of incidence. Several examples of different angles of incidence and of reflection are shown in the diagram. The angle is calculated from the perpendicular (90 degrees; also called the right angle).

4.

If the angle of incidence is ninety degrees, then the angle of reflection is also ninety degrees and the light ray will propagate back along the same path as the incoming light ray (see light ray ‘d’).

1.

It becomes even more interesting, when the surface of the mirror is not flat but curved. An example of such a mirror is a parabola (see diagram).

2.

If the parabola is concave (con = with; cave = space inside) then incoming parallel light rays will reflect back towards a point. This point is called a focus or a focal point.

3.

The distance between the surface of the mirror and the focal point is called the focal length.

4.

The light rays reflecting from the parabola to the focus are converging (=coming close to each other) (diagram B).

5.

The light rays converging to the focal point do not stop there but continue their radiation; they now diverge from this focal point (= diverging).

6.

If the parabola is strongly curved, then the focal distance (=focal length) will be very short. If the parabola is slightly curved, then the focal point will be further away (diagram C and D).

7.

If the parabola is not concave, but convex, then the opposite happens: the light rays are bounced back and diverge from the mirror. They do that with the same mathematical precision as with the concave mirror; the angle of reflection is always equal to the angle of incidence.

8.

Therefore, a convex parabola has also a focal point (dashed in the diagram). But this focal point is not real but imaginary; also called virtual.

1.

If the light rays propagate through a transparent object, then it is not reflected but ‘broken’, bent, refracted.

2.

The refraction occurs at the surface between these two media. The degree (the amount) of refraction (= degree of bending) depends on the density of the two media it passes through.

3.

A convex lens is a good example of refraction. Parallel light rays travelling through a lens are broken.

4.

A light ray that propagates exactly through the centre of a lens is not broken as its angle of incidence (90 degrees) = angle of refraction (also 90 degrees). The same principle as with the mirror. (See point 4 in the Reflection paragraph)

5.

Light rays that propagate off the center get refracted more and more according to the increase in curvature along the lens. All these light rays converge to one point: the focal point.

6.

If the lens is strong (= has a lot of curvature), then the refraction will be more, and the focal point will be close to the lens. But if the lens is weak, then the refraction will be less and the focal point moves further away.

7.

In a concave lens, the light rays will not converge but diverge as they come out of the lens. And they will diverge depending on the degree of curvature as if they originated from a virtual focal point (diagram).

8.

Lenses are very important in medicine as they are used in correcting the vision of patients (spectacles). A convex lens is also called a positive lens; a concave lens is called a negative lens.

1.

The strength of a lens (the strength of refraction) is expressed in diopters (unit = D). The definition of one diopter is a focal length of 1 meter.

2.

If the focal length becomes shorter, due to a stronger lens, then the diopter value increases. So, the formula is the reciprocal (= opposite) of the focal length (see diagram).